1) Three point charges are placed at the vertices of equilateral triangle with sides d. The charges are all positive and of magnitude Q.

a) What is the force on each charge?

b) What is the electric field at the centre of the triangle?

c) What is the electric field at the mid-point of the base of triangle?

2) Three charges are placed along a straight line at positions -d, 0, and +2d. If the charges have the values -0.2Q, Q, and q, what must be the ratio q/Q in order for the net force on charge Q to be zero?

3) The figures below show two configurations of 2 positive and 2 negative charges with separations of order d. These configurations, with net charge of zero and no dipole moment are called quadrupoles. Quadrupole distibutions of charge are important in some focussing and trapping situations as well as very interesting probes of the shapes of nuclei.

a) For each configuration sketch the electric field both close to the charges (out to about 2-3d) and very far from the charges (out at >10d). (That is four sketches.)

b) Compare the two sets of figures and comment on differences and similarities.

4) Book problem 22-51.

Two large parallel copper plates are a distance d apart and have a uniform electric field between them (Figure). An electron is released from the surface of the negative plate at the same time that a proton is released from the positive plate. Neglecting the force of interaction between the particles, find their distance from the positive plate when the particles pass each other.

Why do we not need to know the electric field to answer this question?

4) Book problem 22-86.

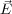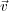In the figure below, a uniform upward electric field of magnitude 2.00 × 10³N/C has been set up between two horizontal plates by charging the lower plate positively and the upper plate negatively. The plates have length L = 10.0cm and separation d = 2.00cm. An electron is then shot between the plates from the left edge of the lower plate. The initial velocity ₀ of the electron makes an angle θ = 45^∘ with the lower plate and has a magnitude of 6.00 × 10⁶m/s.

a) Will the electron strike one of the plates?

b) If so, which plate and how far horizontally from the left edge will the electron strike?

6) Book problem 24-22.

In the figure below a particle of charge +e is initially at the coordinate z = 20nm on the dipole axis, on the positive side of the dipole. (The origin of z is at the dipole center.) The particle is then moved along a circular path around the dipole center until it is at coordinate z = -20nm. The graph gives the work, W_a, done by the force moving the particle versus the angle θ that locates the particle. The scale is set by W_as = 4.0×10^-3J. What is the magnitude of the dipole moment?

7) Book problem 24-50 (altered).

A thin, spherical, conducting shell of radius R is mounted on an insulating support and charged to a potential -V ₀. An electron is then fired directly toward the center of the shell from a point P at a distance r ≫ R. What initial speed, v₀, is needed for the electron to just reach the shell before reversing direction?

8) Three equal charges, Q, are placed at the corners of an equilateral triangle of side d. There is a charge -q at the centroid of the triangle.

a) Compute the total energy needed to construct this system.

b) If the centre charge and the centroid of the triangle are held fixed, find the value of q needed to make the net force on each of the charges Q zero (so the system is in equilibrium).

c) Is the resulting system stable or unstable to small changes in d? If it is unstable, what is the ultimate fate of the system?

9) Consider two metal spheres, A and B, that rest on insulating support posts so that they can be moved around without altering the amount of charge on a sphere. We start with the spheres a long way apart (>>radius of 1 sphere) and put some amount of negative charge on sphere A while leaving B neutral.

a) So long as the spheres are a very long way apart what can you say about the distribution of charge on A (a picture would be good here!).

b) The two spheres are brought closer until they are a few radii apart. Now sketch the approximate distribution of charges on the spheres (remember that A still has a net charge while B is still neutral).

c) A small pith ball, covered in aluminium paint and suspended from an insulating string, is now brought up, suspended between the two spheres, and held in place. It is then released. Once released it moves rapidly to touch one sphere then dashes off to hit the other sphere, back to the first sphere and so on. This goes on for a few seconds and then the ball stops hitting the spheres and just swings to rest like any other underdamped oscillator.
Explain in detail, with good step-by-step diagrams, why the ball swings at first and then explain why it eventually stops being driven. Since the ball is initially electrically neutral you must justify the initial force on the ball particularly carefully.

10) a) Sketch the electric field around a single +ve point charge.

b) Sketch the electric field around two nearby +ve point charges. You should make sure that you make clear the 3-D arrangement of the field, possibly by drawing the field from different angles.

c) Sketch the electric field around 3 colinear, equally spaced, +ve charges.

d) Based upon these findings, discuss (with figures) the field around a long line or rod with a uniform charge density along its length.