Basic Facts 1
This is the first of a weekly set of web pages that sumarize the material of the course. Material appearing on these pages is likely to appear in the weekly quizzes. You are responsible for commiting the material on these pages to memory.
Physical Measurements and Units
We shall work with the following physical quantites in terms of these units.
Quantity |
Unit |
Dimensions |
Meaning |
Mass |
kilogrammes (kg) |
kg |
amount of material |
Length |
metres (m) |
m |
|
Time |
seconds (s) |
s |
|
Temperature |
Kelvin (K) |
1K = 1°Celsius |
|
Velocity |
metres per second |
m s-1 |
how fast something goes |
Acceleration |
metres per second squared |
m s-2 |
how fast velocity alters |
Force |
Newtons (N) |
1N = 1 kg m s-2 |
a push or pull, what it takes to make something
accelerate |
Work |
Joules (J) |
1J = 1 kg m2 s-2 |
force times distance, when you do physical work you get
tired |
Energy |
Joules (J) |
1J = 1 kg m2 s-2 |
Energy is a measure of the ability to do work. |
Pressure |
Pascals (Pa) |
1 Pa = 1 N m-2 |
Force per unit area. Used to describe forces that are
applied over some large area. Force = Pressure × Area |
Remember that distance, velocity, acceleration, and force are all quantities that have both a size and direction associated with them. We call quantities with both a size and a direction VECTOR quantities.
The other quantities, mass, time, temperature, work, energy, and pressure all have only a size. Quantities that have only a size are called SCALAR quantities.
Number Representations and Power Names
We shall find it useful to use Scientific Notation to write out numbers. The significant digits will be written starting in the tens or units place and with as many decimal places as are waranted by the precision of the number. The actual magnitude will then be added by showing a power of ten multiplier. For example
125,536 = 1.2536×105, 100,000,000 = 108, 0.013 = 1.3×10-2, 0.000001156 = 1.1156×10-6.
We often simplify our use of scientific notation by the use of power names. Thus we say 1000m = 1kilometre and 0.001m = 1millimetre. Here are the common prefixes in use
Prefix |
Symbol |
Power |
Example |
mega |
M |
106 |
1,000,000 Joules = 1 mega Joule = 1 MJ |
kilo |
k |
103 |
1,000 metres = 1 kilometer = 1 km |
milli |
m |
10-3 |
0.001 grammes = 1 milligramme = 1mg |
micro |
µ |
10-6 |
0.000001 Newtons = 1 micro Newton = 1µN |
NOTE. No matter what Christopher Loyd ("Doc" in Back to the Future) thinks, giga is pronounced geega not djiga!
Periodic Motion
Periodic motion is any motion that repeats itself after a time
called the Period. symbol P or τ.
We define the Frequency of motion by f = 1/τ so that τ = 1
/f.
We measure the frequency in Hz. 1 Hz = 1 repeat per second.
An oscillating system (and this includes a system that is
to transmit a wave) must have
1) a mass--a source of inertia.
When the mass moves it carries energy in the form of Kinetic
Energy.
2) a restoring force--a force like a spring that pulls back when
pushed and pushes back when pulled.
When the "spring" is compressed or expanded it stores energy in the
form of Potential Energy.
A simple oscillator moves in a way that we call Sinusoidal
Motion.
A graph of the position of the mass versus time looks like this
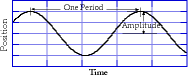
NOTE. We also sometimes use the term Simple Harmonic Motion.
Useful Facts
These merit inclusion but are not required to be memorized.
More Units
These are prefixes that you may meet but that are less common. You should be aware of them and know where to look them up but you don't have to have them memorized.
Prefix |
Symbol |
Power |
Example |
tera |
T |
1012 |
1,000,000,000,000 Watts = 1 Terawatt = 1 TW |
giga |
G |
109 |
1,000,000,000 Pascals = 1 giga Pascal = 1 GPa |
centi |
c |
10-2 |
0.01 metres = 1 centimetre = 1cm |
nano |
n |
10-9 |
0.000000001 Joules = 1 nano Joule = 1nJ |
pico |
p |
10-12 |
0.000000000001 m = 1 pico meter= 1 pm |
Angular Frequency
We often use a scaled version of frequency becuase it fits the math better. The angular frequency has symbol ω, the Greek letter omega. We have ω=2πf.
